The Gap Between Policy and Practice: Where Textbooks Fail Teachers

Guest author William Schmidt, university distinguished professor and founder and director of the Center for the Study of Curriculum Policy at Michigan State University and Shanker Institute Board member, discusses how mathematics education must provide all children not only the formal ideas, concepts, algorithms, and procedures that define mathematics, but also focus on opportunities to experience quantitative reasoning to solve higher-order real-world applications.

The world in which we now live has become increasingly complicated, not just in terms of artificial intelligence (AI), computers, robotics and other forms of technology, but in terms of the ways in which we acquire the knowledge we need to live, work and respond to the complicated issues that now confront the world’s population. Pandemics rage, economies plunge, and the occurrence of floods, hurricanes, tornadoes, and earthquakes increase exponentially in conjunction with climate change. Understanding these issues not only requires literacy in the sense of being able to comprehend what you read, but also requires mathematics literacy, such that a person is able to comprehend the necessary information that increasingly is numerical in nature and is often presented in graphical or tabular form.

Such data also require a background surrounding the following mathematics topics: fractions, rates, ratios, percentages and proportionality, that need to be interpreted correctly to understand the given results. Nothing illustrates the need for such mathematics literacy more than the current stages of the COVID-19 pandemic.

Data are presented daily with respect to the number of cases, the number of hospitalizations, the number of deaths, and the number of vaccinations for different countries, regions, and cities. People look to see how their region compares to others or use it to make decisions about whether to get vaccinated, when to wear a mask, or where to travel.

To effectively use this information, a person must understand what the denominator of the percentage is to make reasoned comparisons among issues involving occurrences, medicines,  deaths, etc. Mathematics literacy also includes knowledge of statistical procedures and statistical reasoning (based primarily on probability) that is increasingly more important in making informed decisions related to both the world of work as well as to personal decisions about health, family finances, schooling options, and filing tax returns. It is also relevant to societal issues such as climate change, inflation rates, income tax policies and country budgets.

For example, those who refuse to get vaccinated because they believe it is dangerous due to possible side-effects often fail to take into account that not getting the vaccination is not free of risk either. The more thoughtful response would be for the person to compare the probability of each. It is this type of statistical reasoning – a key component of mathematics literacy – that must be provided to all children by their schooling no matter their socio-economic status.

Mathematics education must continue to provide all children with the formal ideas, concepts, algorithms and procedures that define formal mathematics, but also focus on providing students opportunities to experience quantitative reasoning (including mathematics, statistics, geometric, and algorithmic reasoning) in the solution of higher-order real-world applications. Perhaps then, we would no longer hear our children say, “Why do I have to learn math? I’m never going to use it!”

In the case of real-world applications such as in the following example, the solution requires, first, the recognition of what the nature of the problem is. Secondly, through the use of mathematics reasoning determine which of the provided information is relevant, and finally, the last step is to set up the problem mathematically and solve it. In many ways this last step is often the least demanding.

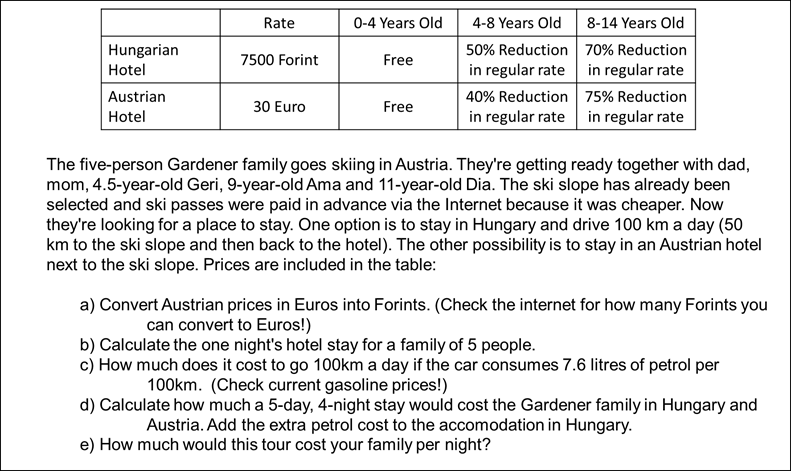
Higher-order real-world applications (HoRw) involve not only the specifically required numbers you need to find the solution as is typical with word problems. They simulate the real-world, messier context in which there is a plethora of quantitative information from which to choose. The problems can even have different solutions depending on the assumptions made. Part of the core task associated with the exercise is to discern what is relevant and what is not and how to mathematically formulate the problem thus leading to a solution.

Opportunities to learn mathematics are clearly related to the types of exercises provided in student textbooks. Teachers are generally not expected to develop their own exercises but to rely on the textbook. Studies indicate that most do as was the case in these 19 countries. The following quote by education psychologists is relevant here as it talks about engaging students in building connections within a domain of knowledge by “…providing opportunities for students to engage repeatedly in this process over time as they deepen and extend their domain knowledge [mathematics literacy]” (Fries et al., 2020, p. 16, emphasis added by blog author).

Textbook data related to the availability of higher-order real-world application exercises in country textbooks paint a dismal picture at best in a 19-country study. The study was done by the Center for the Study for Curriculum Policy at Michigan State University in conjunction with the Organization for Economic Co-operation and Development (OECD).

From the point-of-view of the students, such opportunities to learn and develop mathematics literacy through higher-order real-world applications are so few so as to be almost nonexistent averaging less than 6 exercises per 8th grade textbook. For the US the average of the two textbooks included in the study was 7.

Across the 23 textbooks from the 19 countries, approximately 81% of the textbook exercises were computational in nature with the other 16% being typical word problems that can be characterized by nothing more than computational problems surrounded by words as can be observed in the following figure, the higher-order real-world applications represent less than 1%.

Pollack, some 40 years ago, looked at the issue of how to teach the application of mathematics to the real-world. What he recommended, essentially, was the use of what we have defined as higher-order real-world applications. He noted that typical word problems are “silly, redundant and even stupid” (Pollak, 1969, p. 17). Pollack also indicated that many times the typical word problems around which the exercises are developed make assumptions that are incorrect and inconsistent with the reality that the item represents.

It is here where the gap between policy and practice occurs as we found very few higher-order real-world application exercises in any of the countries textbooks. They occurred in such small numbers that the average country would run out of such exercises in one and a half months even if they only included one such exercise per week.

The results of this study indicate that the countries’ education policy makers have “talked the talk” in terms of the importance of providing opportunities to all students to develop mathematics literacy that enables them to reason mathematically toward finding solutions to the problems that arise in their current lives and certainly almost daily in their future lives as citizens.

Unfortunately, this leaves countries, including the US, with strong policy but weak implementation. Countries do not publish textbooks but rely on the private sector for their development, however, this does not imply that the policy makers should have no influence over the content of the textbooks. Perhaps newly developed policies could impact the inclusion of higher-order real-world applications in mathematics textbooks.

Without such, one can only imagine the difficulties in reaching the important goal of providing all children, no matter their socio-economic status the opportunity to acquire the second most important literacy – mathematics. Without this, we are depriving many students of the opportunity to develop the mathematics knowledge and quantitative reasoning necessary to, as a society, address the serious problems facing the world including climate change, raging pandemics, and even the very survival of democracy.